All engineering surfaces exhibit some level of microscopic roughness. The resistance to heat flow through a contact interface occurs because only a small portion (usually 1-2%) [1] of the nominal surface area is actually in contact. Heat may pass through the interface via three paths: conduction through the contact spots, conduction through the gas present in the gap between the surfaces, and radiation across the gap. Convection may be neglected due to the small length scales involved. Also, radiation does not play a significant role at temperatures below 500°C [2].
Since the conductivity of the gas is much smaller than that of the substrate, most of the heat is constrained to flow through the contact spots. This constriction and subsequent spreading of heat flow lines in the two materials in contact manifests as a thermal resistance at the interface. The total contact resistance of the surface is found by summing, in parallel, the constriction resistances of all of the contact spots.
The prediction of thermal contact conductance (inverse of resistance) is commonly accomplished by semi-empirical correlations. With assumptions of a particular statistical distribution of surface parameters, as well as a mode of deformation for contacting asperities, contact conductance is predicted for given material properties and applied load [3]. Since the surface parameters, such as asperity height, density, slope, and radius of curvature, are not intrinsic properties of the surface, but instead can vary with the sampling frequency, the contact conductance predicted from these equations is dependent on the scanning instrument used.
More recent attempts at predicting thermal contact resistance at an interface, especially focusing on the low contact pressures allowable in electronics cooling, have taken advantage of the speed and processing power of modern computers to track the number of asperities in contact at a surface by directly using surface profile data [4]. Inputs to the model [4] include the surface profiles, mechanical and thermal material properties, nominal contact area, and specified loads. The mode of deformation of each asperity is not assumed, but determined by guessing the mean plane separation between the surfaces, calculating the pressure and area of each microscopic asperity in contact, and summing the contribution to the total load from each asperity.
This iterative process is repeated at various assumed values of mean plane separation until the sum of the load contributions from individual asperities is within some tolerance of the specified nominal load. Once the deformation, and hence the geometry, of each asperity is known for a given contact pressure, the contact resistance may be calculated by summing all of the constriction resistances. One recent constriction resistance model [5] uses an improved geometrical shape for the asperities in contact. The resulting correlation takes into account the gas gap conductivity and is readily implemented in a computer algorithm.
The models described in [4] and [5] can be used in combination to predict the contact conductance at any surface. However, as with the semi-empirical correlation method, results from this prediction scheme are dependent on details of the number of asperities calculated and their geometry, which in turn is a function of the resolution of the scanning instrument used for surface characterization.
This dependence may be handled by functional filtering, in which the data are filtered to the proper wavelengths involved in the physics of deformation. The long-wavelength cutoff is easily determined from the nominal area of contact, but there is no equally obvious choice for the short-wavelength cutoff. The short-wavelength cutoff is likely to be a function of the load and material properties, and of intrinsic surface properties, which are independent of the scanning instrument.
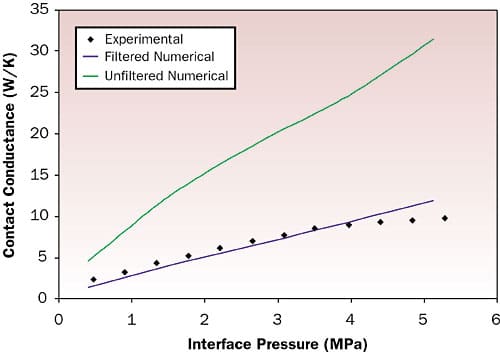 |
Figure 1. Comparison of predictions to experimental measurements of contact conductance. The results are for contact between two aluminum surfaces with a 5.0 centerline average surface roughness. The unfiltered data were taken with a sampling frequency of 640/mm and the filtered data has a low-pass cutoff wavelength of 19 �m.
One technique for finding such intrinsic surface properties [6] involves using the structure function to characterize a surface. For a multi-fractal surface the transition between modes of surface preparation are marked by a particular frequency. Predictions for contact conductance are obtained by passing the input profiles through a low-pass filter with a cutoff frequency equal to the transition frequency, and by using the computer algorithm already discussed. Results from the prediction using filtered and unfiltered surface profiles appear in Figure 1. The experimental measurements in Figure 1 were obtained using the axial heat flow facility shown in Figure 2.
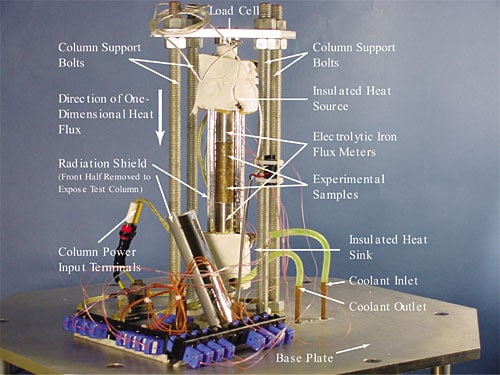 |
Figure 2. Axial heat flow test facility used in the measurement of contact conductance.
The approach described has been successful in predicting contact conductance at the interface between metallic surfaces, as validated by comparison with experimental measurements. The current results are applicable, in particular, under situations where metal to metal contacts are encountered at high heat fluxes (RF transistors, power electronics components, and others). Extensions to the model for handling non-metallic materials, more complex non-flat surface contact, and the presence of thermal interface materials, are in progress.
References
- Bowden, F.P. and Tabor, D., The Friction and Lubrication of Solids, Oxford University Press, London, 1950, pp. 20-32.
- Olsen, E.L., Garimella, S.V., and Madhusudana, C.V., “Modeling of Constriction Resistance in Coated Joints,” AIAA Journal of Thermophysics and Heat Transfer, Vol. 16, 2002, pp. 207-216.
- Sridhar, M.R. and Yovanovich, M.M., “Elasotplastic Contact Conductance Model for Isotropic, Conforming Rough Surfaces and Comparison with Experiments,” Journal of Heat Transfer, 1996, Vol. 118, pp. 3-9.
- Singhal, V. and Garimella, S.V. “Prediction of Thermal Contact Conductance by Surface Deformation Analysis,” Proceedings of International Mechanical Engineering Congress and Exposition, New York, IMECE2001/HTD-24376, pp. 1-8, November 2001.
- Black, A.F., Singhal, V. and Garimella, S.V., “Analysis and Prediction of Constriction Resistance for Contact between Rough Engineering Surfaces,” AIAA Journal of Thermophysics and Heat Transfer (in press).
- Thomas, T.R., Rough Surfaces, Imperial College Press, London, 1999, pp. 151-168.










