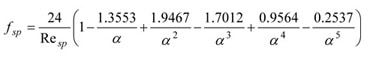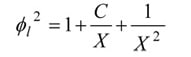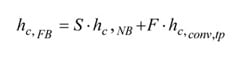Small-channel heat sinks provide an extremely compact and efficient vehicle for dissipation of large heat fluxes typically found in high power electronics. Fluid flow and heat transfer in small-sized channels, with hydraulic diameters on the order of a fraction of a millimeter (a few hundred micrometers), have been shown to behave similarly to conventional-sized channels (hydraulic diameter of many millimeters) for single phase liquid flow. Many studies have established that the classical behavior, as predicted by Navier-Stokes equations, remains valid for small channels [1-3] for single phase liquid flow. However, a departure in small channel two-phase flowbehavior has been observed from that of conventional-sized channels. A significant amount of work has been dedicated to measuring and predicting the heat transfer behavior in small-channel heat sinks for two-phase flow [6-14]. Each flow configuration, single-phase or two-phase flow, comes with its unique advantages and challenges. This article presents a case study to outline the advantages and challenges, and presents a systematic methodology for the calculation of fluid flow and heat transfer parameters for each flow configuration for small-channel heat sinks.
Theory and Modeling
Single Phase Flow
The physics of single-phase flow and heat transfer is well understood and has been substantiated over the years. It has been shown conclusively in the literature that it remains applicable to channels that are much smaller in diameter than the conventional channels encountered in typical coldplate applications. For single-phase laminar liquid flow in small channels, the frictional pressure drop for hydrodynamically developed flow can be expressed as follows:
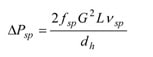 |
(1)
Where the friction factor f sp can be expressed as [4],
(2)
The Nusselt number for thermally fully developed laminar flow in a channel heated on three sides is given as [5]:
| |
(3)
Equations (1) to (3) complete the definition required to calculate the pressure drop and heat transfer coefficient for fully developed single-phase flow in small channels.
Two-Phase Flow
The physics of two-phase flow and heat transfer is more complex. The traditional approach has been to utilize the knowledge of the single-flow physics and modify it based on experimental correlations and theory to derive semi-empirical correlations. These correlations can be used to provide two-phase flow behavior. The general method for pressure drop calculation has been to compute the pressure drop for the liquid phase flow and modify it using a pressure drop multiplier in the following manner:
|
|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||
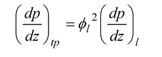 |
(4)
The pressure drop calculation in Equation (4) still utilizes Equation (1) but with one difference. While the single-phase subscript sp in Equation (1) implies the calculation for the liquid phase flow for the flow comprised entirely of the liquid, the subscript l in Equation (4) refers to the pressure drop calculation attributable to just the liquid phase portion of the two phases that exist simultaneously. Hence, the mass flux, G, is scaled by the mixture quality to compute the single-phase pressure drop in the liquid or the vapor phase as listed in Equations (5) and (6). This leads to the following definitions:
 |
(5)
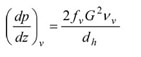 |
(6)
to the total mass of the mixture, mathematically defined as:
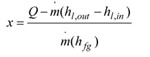 |
(7)
This approach was pioneered by the work performed by Lockhart and Martinelli [6], and although a number of variations of this approach exist, this basic methodology is consistent in a vast majority of the published work, including [8-10] and [12-14]. Numerous ways have been proposed in the literature for the calculation of the two phase pressure drop multiplier, Φ2. A traditional form of the two-phase multiplier is:
(8)
where C is a parameter proposed by Chisholm [8] and is a function of liquid and vapor flow regimes. This article will utilize the methodology proposed by Sun and Mishima [9] for the determination of the two-phase pressure drop multiplier. They proposed a new form of the Chisholm parameter, C, for laminar flow and showed it to fit a large amount of experimental data from various studies:
 |
(9)
The Laplace number, La, is a measure of the surface tension and buoyancy effects:
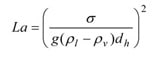 |
(10)
Also, X, the Martinelli parameter is a ratio of the liquid phase pressure drop to the vapor phase pressure drop as follows [6]:
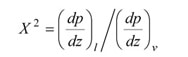 |
(11)
which makes the Martinelli parameter, X, a known parameter for a given flow condition. Equations [4-11] complete the definition for pressure drop in two-phase flow.
The prediction of heat transfer in two-phase flow is challenging because of the simultaneous existence of the liquid and vapor phase convective heat transfer as well as the boiling heat transfer. Several approaches exist — some that rely mostly on boiling heat transfer and many others that consider the effect of convective as well as boiling heat transfer. One particular approach that accounts for both effects, and will be demonstrated in this article, is of the form [7]:
(12)
where S is a suppression factor for the nucleate boiling term as additional liquid is converted to vapor during the boiling process and F is the enhancement factor to account for the increased rate of convective heat transfer as flow velocities increase due to the larger specific volume of the vapor phase. Several ways have been proposed in the literature for the calculation of the suppression and enhancement factors, S and F, and the heat transfer coefficients related to nucleate boiling and two-phase convection. This article will demonstrate the one proposed by Bertsch, Groll, and Garimella [10] for the determination of the heat transfer parameters, including the suppression and enhancement factors and heat transfer coefficients.
References [9] and [10] were chosen for pressure drop and heat transfer calculations, respectively, since they are recent and have compared their methodology against a comprehensive database of experimental and empirical predictive work. It should be noted that since two-phase flow is not well understood, any particular set of correlations from a published study may be prone to errors under certain conditions. Consequently, reliance on any one particularstudy is not recommended; however, a detailed examination of any single study reveals the underlying physics. The knowledge acquired, however, can be used to formulate the analysis methodology for a real application.
Bertsch et al [10] proposed employing Cooper’s [11] pool boiling correlation for the nucleate boiling term, h, given as:
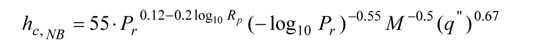 (13) (13) |
For the convective term, h, they proposed the following:
| |
(14)
In other words, the contribution to the convective two phase flow was proportioned between the liquid phase, hc,conv,l, and the vapor phase, hc,conv,v, in proportion to the mixture quality level, x. Hausen’s correlation [15] was suggested for the determination of liquid and vapor phase heat transfer coefficients. The proposed suppression factor, S, is (1-x), while the resulting enhancement factor, F, was derived from fitting a curve to a large database as: [1+80(x2 —x6)e-0.6La]. This resulted in the heat transfer coefficient for the two-phase flow of the form [10]:
| |
(15)
Equations (1) to (15) complete the definition of single-phase (liquid) and two-phase pressure drop and heat transfer for the purpose of this article. Their application is being demonstrated in the next section.
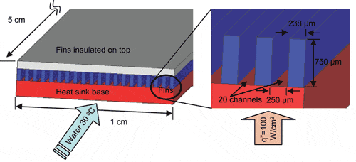
A Representative Application of Liquid and Two-Phase Cooling
A small heat sink, 1cm wide and 5 cm long, was chosen for illustration purposes. The configuration of the heat sink and the microchannels is shown in Figure 1. The choice of this particular configuration was motivated by published studies by Mudawar et al [12, 13] for which the experimental data is also available. The heat sink had 20 machined channels that are each 750 µm tall and 250 µm wide. The top of the channels were insulated, which resulted in three-sided heating of the channel. Fin efficiency calculations showed that these fins were approximately 90% efficient at the design conditions for both the liquid and the two-phase flow. As expected, due to the lower heat transfer coefficient, single-phase flow resulted in slightly higher fin efficiency. For simplicity in the analysis, the fin efficiency was held constant at 90%. Water was used as the working fluid for this demonstration. An inlet temperature of 30oC was used for both the single and two-phase cooling. All analysis was conducted for a heat sink base heat flux of 100 W/cm2. The analysis parameters are shown in Table 1.
Single Phase Pressure Loss and Heat Transfer
Equations (1) and (2) were used to determine the pressure drop in the heat sink shown in Figure 1 for the parameters shown in Table 1. A mass flux of 1150 kg/m2-s (or 4.3e-3 kg/s) was chosen to maintain the liquid in single phase at the exit of the heat sink. Fluid properties were calculated at the mean of the inlet and the outlet temperature.
Calculations show that the flow is laminar with a Reynolds number of 675. It is hydrodynamically developed and thermally developing at the heat sink exit. A frictional pressure loss of 9520 Pa (or 1.38 psi) was computed using Equations (1) and (2). In addition to the frictional pressure loss, the other mechanisms that result in pressure loss are due to acceleration, contraction, and expansion. Accelerational pressure loss is due to an increase in the liquid specific volume as its temperature rises along the channel length. It was negligible for this case study. Contraction pressure loss results from the fluid being funneled into the heat sink from a larger opening at the entrance. The entrance region was assumed to be the same size as the total heat sink cross-sectional area, 1 cm wide by 750 µm high. This resulted in a flow contraction ratio of 0.5, i.e. half the flow volume was occupied by the fin walls in the heat sink volumetric space. This contraction pressure drop loss computed to be about 1200 Pa (or 0.17 psi). The final term is the pressure recovery at the exit when the liquid expands from a smaller volume (channels) into the exit manifold. The pressure recovery was computed to be 423 Pa (or 0.06 psi). The reader is encouraged to refer to [14] for more information on contraction pressure losses and expansion recovery.
 (16) (16) |
Hence, the total pressure loss was computed to be 10297 Pa (or 1.49 psi), with approximately 92% associated with the frictional pressure loss.
Equation (3), for 3-sided heating of a channel, was used to determine the Nusselt number for the liquid flow. The computed average Nusselt number for the channel was 5.82, resulting in a heat transfer coefficient, computed ash =Nu k/d, of 10090 W/m2-K. This heat transfer coefficient results in a heat sink base temperature rise of 31oC above the cooling liquid temperature. It should also be noted that there is considerable temperature gradient along the heat sink base, from the inlet to the exit due to the fluid heating along the length of the heat sink.
Two- Phase Pressure Loss and Heat Transfer
This simulation was similar to the single-phase conditions except that the flow rate was reduced to ensure that a two-phase condition existed for a significant portion of the channel along the heat sink length. A mass flux was chosen which resulted in nearly the same pressure loss as the single-phase case, equal to approximately 10,000 Pa.
|
Table 1. Analysis parameters for this case study
A mass flux of 150 kg/m2-s (or 5.6e-4 kg/s) was used. This resulted in single phase liquid condition in one-third of the channel length, or
15.7 mm, and two-phase in the remaining two-thirds, or 34.3 mm. A set of inlet conditions and mass flow rate could be chosen to create two-phase conditions along the entire length of the channel, if so desired. The following contributions from the various pressure drop mechanisms were found by using equations (1-2) and (4-11) for pressure drop in single and two-phase flow:
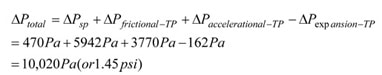 |
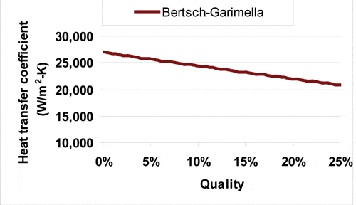
Equation (15) predicts a heat transfer coefficient that is a function of mixture quality, or effectively, the position along the length of the channel as the mixture quality changes. The variation of heat transfer coefficient with quality is shown in Figure 2. Heat transfer coefficients ranging from 20,000 to 27,000 W/m2-K were achieved in the two phase region, which results in heat sink-to-fluid temperature differences between 11.6 and 15.6oC. The larger sink-to-fluid temperature difference occurs at the heat sink exit due to the degradation in heat transfer coefficient with increasing quality along the channel length.
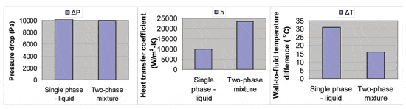
A comparison of the heat sink performance for the single-phase and two-phase flow conditions is shown in Figure 3. The values plotted for two-phase flow are at the center of the channel. The plots in Figure 3 show that the average two-phase flow heat transfer coefficient of 23,000 W/m2-K is more than twice the single phase flow configuration (10090 W/m2-K), at a similar pressure drop of about 10,000 Pa (1.5 psi) for each configuration. Additionally, the enhanced heat transfer coefficient from two-phase flow results in a substantially lower wall-to-fluid temperature difference: an average of 13.6oC as compared to 31oC for single phase flow. One other key discriminator between the two cooling schemes is that the saturation state in two-phase flow will maintain a nearly constant fluid and heat sink wall temperature, versus single-phase flow where the fluid rises in temperature along the length of the heat sink.
Conclusions
This case study presents a systematic study of the calculation of, and tradeoffs between, single and two-phase cooling schemes. The pressure loss and heat transfer coefficients were compared for each cooling scheme. The data presented herein demonstrates that while single-phase and two-phase cooling are both viable options for cooling applications with high heat fluxes, two-phase cooling provides enhanced heat transfer at the same system pressure loss.
References
- Liu, D., and Garimella, S.V., “Investigation of Liquid Flow in Microchannels,” AIAA Journal of Thermophysics, Heat Transfer, 18, 2004, pp. 65-72.
- Lee, P.S., Garimella, S.V., and Liu, D., “Investigation of Heat Transfer in Rectangular Microchannels,” International Journal of Heat and Mass Transfer, 48, 2005, pp. 1688-1704.
- Judy, J., Maynes, D., and Webb, B.W., “Characterization of Frictional Pressure Drop for Liquid Flows Through Microchannels,” International Journal of Heat and Mass Transfer, 45, 2002, pp. 3477-3489.
- Shah, R.K. and London, A.L., “Laminar Flow Forced Convection in Ducts,” Supplement 1 to Advances in Heat Transfer, New York: Academic Press, 1978.
- Phillips, R.J., “Forced Convection, Liquid Cooled, Microchannel Heat Sinks,” MS Thesis, Massachusetts Institute of Technology, 1987.
- Lockhart, R.W. and Martinelli, R.C., “Proposed Correlation of Data for Isothermal Two-Phase, Two Component Flow in Pipes,” Chemical Engineering Progress, 45, 1949, pp. 39-48.
- Chen, J.C., “Correlation for Boiling Heat Transfer to Saturated Fluids in Convective Flow,” I&EC Process Design and Development, Vol. 5 (3), 1966, pp. 322-329.
- Chisholm, D., “A Theoretical Basis for the Lockhart-Martinelli Correlation for Two-Phase Flow,” International Journal of Heat and Mass Transfer, 10 (12), 1967, pp. 1767-1778.
- Sun, L. and Mishima, K., “Evaluation Analysis of Prediction Methods for Two-Phase Flow Pressure Drop in Mini-Channels,” International Journal of Multiphase Flow, 35, 2009, pp. 47-54.
- Bertsch, S.S., Groll, E.A., and Garimella, S.V., “A Composite Heat Transfer Correlation for Saturated Flow Boiling in Small Channels,” International Journal of Heat and Mass Transfer, 52, 2009, pp. 2110-2118.
- Cooper, M.G., “Heat Flow Rates in Saturated Nucleate Pool Boiling – A Wide-Ranging Examination Using Reduced Properties,” Advances in Heat Transfer, V. 16, 1984, pp. 157-239.
- Qu, W. and Mudawar, I., “Measurement and Prediction of Pressure Drop in Two-Phase Micro-Channel Heat Sinks,” International Journal of Heat and Mass Transfer, 46, 2003, pp. 2737-2753.
- Qu, W. and Mudawar, I., “Flow Boiling Heat Transfer in Two-Phase Micro-Channel Heat Sinks – I. Experimental Investigation And Assessment of Correlation Methods,” International Journal of Heat and Mass Transfer, 46, 2003, pp. 2755-2771.
- Collier, J.G. and Thome, J.R., “Convective Boiling and Condensation,” Third edition, Oxford University Press, Oxford, 1996, pp. 108-112.
- Incropera, F.P. and Dewitt, D.P., “Fundamentals of Heat and Mass Transfer,” Fourth edition, John Wiley and Sons, 1996, pp. 443-444.