In keeping with the name of this column, the fact worth reminding is that a fixed temperature boundary condition in a thermal simulation represents an infinite heat sink. The systems that thermal engineers model and simulate have boundaries and thus require some type of specification, usually known as boundary conditions. Related to the other part of the name of this column, an incorrect boundary condition specification can lead to “fairy tale” predictions.
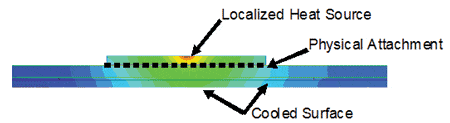
Consider the situation illustrated by temperature contours in Figure 1. At the top of the figure, there is a heat generating object such as a semiconductor die. Between the die and the cooled surface are packaging materials that provide structural, environmental, and thermal functions. These could also represent a heatsink. The physical attachment layer in this case represents the die attach material.
From a physical standpoint, the attachment location divides the heat generating die from the packaging material (heatsink). Frequently, the heat generating die and heatsink are obtained from different companies. Since neither company knows precisely how their products will be integrated together, each company will make assumptions for the appropriate thermal boundary condition at this interface. The potential for problems results in how they treat the thermal boundary at the physical attachment layer (dashed line). It is worth noting that from a physics standpoint, the dashed line doesn’t represent a uniform temperature, its location is defined by the physical geometry.
For a semiconductor die mounted as in Figure 1, the manufacturer may provide a junction to backside thermal resistance. One way of generating this information is to assume that the lower surface of the die is held at a fixed temperature. From looking at temperature isotherm plot in the figure, this is not a valid assumption but the manufacturer does not necessarily know how the die is mounted and a junction to backside thermal calculation done this way is a best case and at least provides a basis of comparison between similar devices. The fixed temperature (infinite heat sink) assumption removes the uncertainty of mounting methods from the manufacturer.
If the heat sink portion of the figure (below the dashed line) was also obtained separately, we would like to know its thermal performance. Assuming that the manufacture made this part for a defined size die, they could arrive at a thermal performance measure based on analysis, test, or both. However, they also need to make an assumption about the thermal boundary conditions at the dashed line and most likely would use a uniform heat flux assumption. The same argument can be made that even though the uniform heat flux assumption is not valid in the application, it allows comparison between similar heat sink packages.
The job of the thermal engineer here is to integrate the thermal performance information from separate manufacturers. In the example above, the heat sink will experience a non-uniform flux and the semiconductor die will experience a non-uniform base temperature. Neglecting these facts will most likely result in a predicted temperature that is lower than actually exists. It is worth noting that the example described is representative of most physical problems that are faced by thermal engineers, namely that the boundary between objects is neither a uniform flux nor a uniform temperature. Using a 1 or 2 resistor thermal representation of an object has an underlying assumption of one-dimensional heat flow which only allows a constant temperature or constant heat flux boundary condition. A more detailed discussion of this topic is found in Reference 1. An additional point worth noting is that a near isothermal object in a simulation or test does not necessarily mean that it may be used as a fixed temperature boundary condition. Using the object as a fixed temperature really means that it temperature will not change under any circumstances, so be sure.
The readers are reminded of the significant progress made in integrating package and system level thermal models by using compact thermal models [2]. These models remove much of the ambiguity associated with understanding the package case temperatures and appropriate thermal flux profiles into the system. However, the goal of this column is a reminder of the need to understand the boundary conditions that are an inherent portion of the manufacturer supplied thermal performance information.
REFERENCES
Lasance, C, “Heat Spreading: Not a Trivial Issue,” ElectronicsCooling, May, 2008.
Shidore, S, “Compact Thermal Modeling in Electronics Design,” ElectronicsCooling, May, 2007.