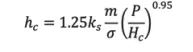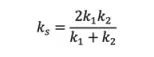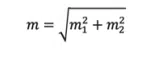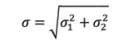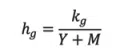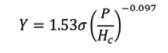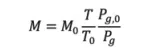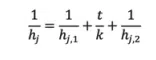Calculating Interface Resistance

Introduction

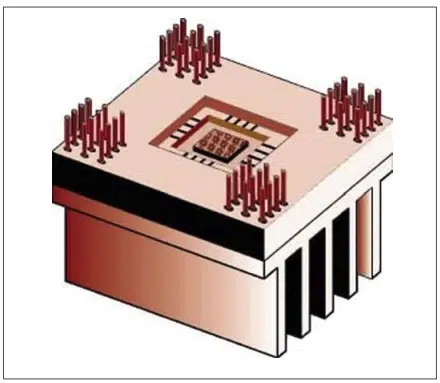
The exposed surface area of many of today’s high-powered electronic packages is no longer sufficient for the removal of the heat generated during normal operation. Heat sinks are a commonly-used, low cost means of increasing the effective surface area for dissipating heat by means of convective air cooling. While the use of a heat sink lowers the fluid-side thermal resistance, it also introduces an interface resistance across the contact formed between itself and the package case. Under some circumstances, this contact resistance can be substantial, impeding heat flow and reducing the overall effectiveness of the heat sink. Figure 1 depicts an electronic package heat sink assembly which would typically be joined by plastic or metal spring clips around the perimeter of the assembly.

The subject of thermal resistance at interfaces between aluminum heat sinks and ceramic packages has been discussed by Lee [1], de Sorgo [2], Latham [3], and Early et al. [4]. These articles primarily report test results for joint resistance as a function of contact pressure for various interface types. The interfaces examined in these works involve either bare surfaces (air filled) or joints where the interstitial gap is filled with a material layer containing dispersed thermally conductive fillers. Interstitial material layers currently used by the industry, as described by de Sorgo [2], include thermal greases, thermally conductive compounds, elastomers, and adhesive tapes.

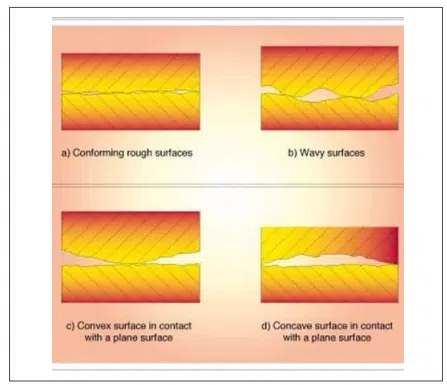
The objective of this article is to illustrate how to calculate the thermal joint resistance for the interface formed by two conforming, rough surface shown in Figure 2a, as a function of contact pressure for the low pressure range, between 0.035 and 0.35 MPa (5 and 50 psi), commonly encountered in microelectronic applications (Latham [3]). Peterson and Fletcher [5] verified by experiments in vacuum that the following models, which were originally developed for metal-to-metal contacts, give very good results when used to predict the contact conductance at interfaces formed by metals (invar, Kovar, and alloy 42) and mold compounds (Polyset 410B and 410C, MG25F-LMP, and MG45F-04) at the interface temperature range: 20°C to 70°C and the interface pressure range: 0.5 to 5.0 MPa. This work will focus primarily on bare joints, although an example where the interface material is treated as a liquid, such as in the case of a thermal grease, will also be considered. Interfaces with thermal compounds or elastomeric sheet materials will be shown to be very difficult to model and will be discussed in general terms only. The non-conforming wavy, convex, or concave interfaces depicted in Figures 2b, 2c, and 2d, respectively, are exceedingly complex to model and therefore will not be considered here. Since radiation heat transfer at most interfaces is negligible or non-existent, it will not be included in this analysis.

Conforming Rough Surface Model

The thermal joint conductance, h_j , of the interface formed by two conforming, rough surfaces is given by the following simple model proposed by Yovanovich [6] and further described and used by Antonetti and Yovanovich [7], Yovanovich and Antonetti [8], and Yovanovich [9].

The contact conductance is given by:

where k_s is the harmonic mean thermal conductivity of the interface:

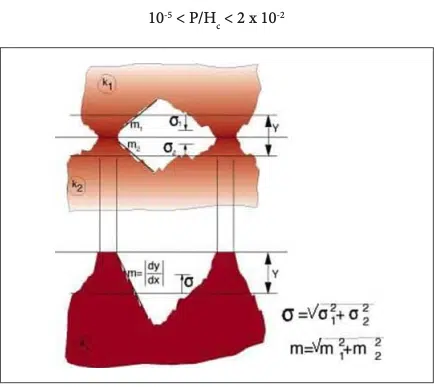
the effective mean absolute asperity slope of the interface m, as shown in Figure 3, is given by:

and where σ, also shown in Figure 3, is the effective RMS surface roughness of the contacting asperities:

The contact pressure is P and H_c is the surface microhardness of the softer of the two contacting solids. The microhardness is in general complex because it depends on several geometric and physical parameters, such as the Vickers microhardness correlation coefficients.

The surface asperity slope is frequently not given. In this case, the mean absolute asperity slope can be approximated by the correlation equation proposed by Antonetti et al. [10]:

which was developed for the surface roughness range:

The gap conductance, h_g , is given by the approximation of Yovanovich [6]:

where k_g is the thermal conductivity of the gap substance. The effective gap thickness Y, shown in Figure 3, can be calculated accurately by means of the simple power-law correlation equation proposed by Antonetti and Yovanovich [7]:

for the relative contact pressure range:

The gas parameter M accounts for rarefaction effects at high temperatures and low gas pressures. This gas-surface parameter depends on the thermal accommodation coefficients, the ratio of specific heats, the Prandtl number, and the molecular mean freepath of the gas. Song and Yovanovich [11] present correlation equations for the calculation of the accommodation coefficients for several gases as a function of the gas temperature. This complex gas-surface parameter depends on gas pressure and temperature according to the relationship:

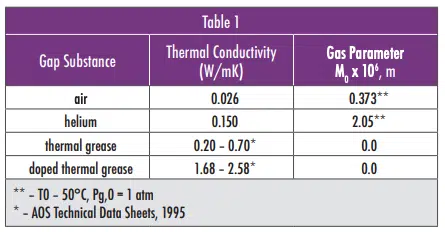
where M₀ denotes the gas parameter value at the reference values of gas temperature and pressure, T₀ and P_g,0, respectively. Reference values of the gas parameter for air and helium are presented in Table 1.

Interstital Material Layers

Although the conforming rough surface model presented in the previous section was developed for bare surfaces, it can also be applied to interfaces with thermal grease. By assuming that the grease behaves as a liquid and fills all gaps between the contacting asperities, the existing model can be used by substituting M = 0 and the thermal conductivity of the grease into the gap conductance relationship. However, when solid interstitial materials are used, such as thermal compounds, elastomers or adhesive tapes, the joint conductance problem becomes much more complicated. As shown in Figure 4, the use of a solid interstitial material introduces an additional interface to the problem.

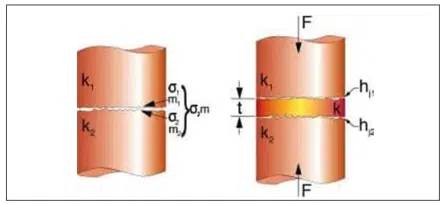
Using thermal resistance concepts, the overall joint conductance for this problem is determined by the series combination:

where h_j,1 and h_j,2 refer to the joint conductance between each of the contacting surfaces and the interfacial material and t and k are the average thickness and thermal conductivity of the layer. Completing this analysis requires characterization of the relevant surface parameters, such as the slope, roughness and microhardness, for the various interstitial materials. In addition, for elastomeric materials the layer thickness t is not constant but instead depends on the contact pressure. Additional research needs to be done before a model can be developed to address this complex phenomenon.

Application to Aluminum Heat Sink- Ceramic Package Interface

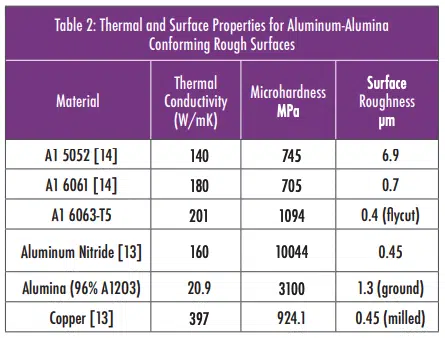
The aforementioned models will be used to calculate the joint resistances for the interface formed by an aluminum 6063-T5 aluminum heat sink and Al₂ O₃ alumina package. The thermal conductivities of the heat sink and ceramic package are k₁ = 201 W/mK and k₂ = 20.9 W/mK respectively. The harmonic mean thermal conductivity of the interface is k_s = 37.85 W/mK. Since the microhardness of the aluminum alloy is 1094 MPa, which is much less than that of the alumina, it will be used to compute the contact parameters. Based on a surface roughness for flycut aluminum of σ₁ = 0.4 µm and a surface roughness for ground alumina of σ₂ = 1.3 µm, the effective surface roughness of the interface is calculated as σ = 1.36 µm. Since the surface slopes are not given, Eq. (6) will be used to calculate the following values: m₁ = 0.139, m₂ = 0.0865, respectively. The effective surface slope of the interface is therefore m = 0.164. The thermal and physical properties of air, helium and grease presented in Table 1 will be used in the gap conductance model.

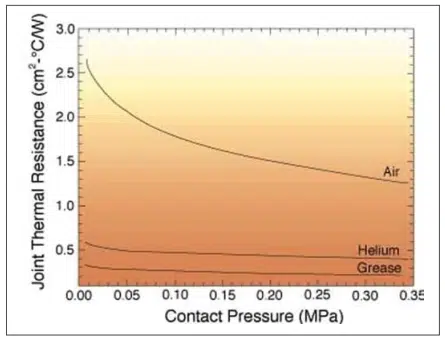
In Figure 5 the joint thermal resistances, whose units are cm² °C/W are plotted against the nominal contact pressure over the pressure range: 0.007 ≤ P (MPa) ≤ 0.35 for several cases. The bare joint resistances with air or helium present in the gap are shown. The effect of a thermal grease of thermal conductivity k_g = 0.20 W/mK is also shown in Figure 5.

Summary and Discussion

Simple correlation equations are presented and used to calculate thermal joint resistances for a typical aluminum-ceramic interface found in microelectronics applications. Flycut and ground surfaces are considered. Joint resistances are calculated for contact pressures between 0.007 and 0.35 MPa, which includes the practical microelectronic pressure range of 0.07 and 0.17 MPa (see Latham [3]). The greatest joint resistances are found when air is present in the interstitial gap. In the contact pressure range of 0.007 to 0.35 MPa, the air joint resistance goes from 2.665 to 1.903 cm² °C/W.

When silicon grease is placed in the gap, the joint resistance is much smaller than the bare interface. The calculated values of the joint resistance lie in the range 0.335 to 0.213 cm² °C/W which are an order of magnitude smaller than the joint resistances of a bare joint. If greases with thermally conductive ceramics are used, the joint resistance can be reduced to values below 0.065 cm² °C/W.

The correlation equations which have been used are based on conforming rough surfaces with interstitial substances which perfectly wet all portions of the surfaces which form the gap. Any non-flatness will result in interfaces with larger gaps which will have larger joint resistances. If the interstitial substance does not perfectly wet the contacting surfaces, this will also produce a more thermally resistive interface. The proposed models and correlation equations therefore correspond to the best thermal joints which have the smallest joint resistances.

The use of other interstitial materials, such as thermal compounds, elastomers or adhesive tapes, has been shown to increase the complexity of the joint conductance problem significantly. In order to successfully model this problem, extensive research into characterizing the surface properties and layer thicknesses for the various interfacial materials is required.

REFERENCES

1. S. Lee, How to Select a Heat Sink, Electronics Cooling, Vol. 1, No. 1, June 1995, pp. 10-14.

2. Miksa de Sorgo, Thermal Interface Materials, Electronics Cooling, Vol. 2, No. 2, September 1996, pp. 12-15.

3. Carol A. Latham, Thermal Resistance of Interface Materials as a Function of Pressure, Electronics Cooling, Vol. 2, No. 2, September 1996, p. 35.

4. Malcolm Early, Seri Lee, and Mark Pellilo, Thermal Performance of Interface Material in Microelectronics Packaging Applications, Proceedings of the 1995 International Electronics Packaging Conference, September 1995, pp. 534-544.

5. G.P. Peterson and L.S. Fletcher, Evaluation of the Thermal Contact Conductance Between Substrate and Mold Compound Materials, ASMEHTD-Vol. 69, Fundamentals of Conduction and Recent Developments in Contact Resistance, edited by M. Imber, G.P. Peterson and M.M. Yovanovich, 1987, pp.99-105.

6. M.M. Yovanovich, New Contact and Gap Correlations for Conforming Rough Surfaces, AIAA-81-1164, presented at AIAA 16th Thermophysics Conference, Palo Alto, CA., June 1981.

7. V.W. Antonetti and M.M. Yovanovich, Thermal Contact Resistance in Microelectronic Equipment, Thermal Management Concepts in Microelectronic Packaging From Component to System, ISHM Technical Monograph Series 6984- 003,1984, pp. 135-151.

8. M.M. Yovanovich and V.W. Antonetti, Application of Thermal Contact Resistance Theory to Electronic Packages, Advances in Thermal Modeling of Electronic Components and Systems, Vol. 1, Editors A.Bar-Cohen and A.D. Kraus, Hemisphere Publishing Corporation, 1988, pp. 79-128.

9. M.M. Yovanovich, Theory and Applications of Constriction and Spreading Resistance Concepts for Microelectronic Thermal Management, Cooling Techniques for Computers, Editor Win Aung, Hemisphere Publishing Corporation, 1991, pp. 277-332.

10. V.W. Antonetti, T.D. Whittle, and R.E. Simons, An Approximate Thermal Contact Conductance Correlation, HTD-Vol. 170, Experimental/Numerical Heat Transfer in Combustion and Phase Change, 1991, pp.35-42.

11. S. Song and M.M. Yovanovich, Correlation of Thermal Accommodation Coefficient for Engineering Surfaces, ASME HTD-Vol. 69, Fundamentals of Conduction and Recent Developments in Contact Resistance, edited by M. Imber, G.P. Peterson and M.M. Yovanovich, 1987, pp. 107-116.