Ed J. Walsh, Jason Stafford & Vanessa Egan
Stokes Research Institute, Dept. of Mechanical and Aeronautical Engineering, University of Limerick
INTRODUCTION
Today’s marketplace consists of many portable computing devices such as personal digital assistants and smart phones. Currently over one billion handset type units are shipped every year. The functionality and power levels within these devices continues to increase causing additional thermal management issues, which will require active cooling solutions in the future. For active cooling in such devices small scale fans will be needed to remove the heat generated, and hence much work needs to be undertaken at this scale[1]. Key issues are cost, acoustics[2], reliability, fan design [3] and cooling efficiency. There are numerous cooling solution designs where fans are placed directly over heat sinks resulting in an impingement type flow field. Currently there is a lack of understanding of the emerging flow field and the resulting local heat transfer rates on the target surface. The influence of motor supports is particularly important for small scale fans as the motor supports do not scale with reducing fan dimensions as can be seen from the small scale fans in the marketplace today. This article demonstrates that the emerging flow and resultant local heat transfer rates from an axial fan are dependent on motor support design and placement. This finding suggests the need for integrated designs of fan-heat sink cooling solutions as suggested previously for radial flow fans [4].
EXPERIMENTAL TECHNIQUE AND FAN
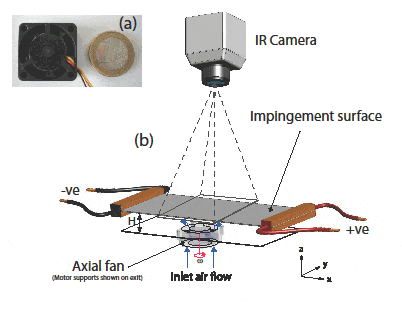
The direct measurement or calculation of local heat transfer coefficient is achieved using the infrared thermography and heated-thin-foil technique. The heated foil is a thin (12.5µm) stainless steel foil that has a constant heat flux boundary condition. The conduction within the foil, radiation and natural convection are accounted for using a technique developed by the current authors [5]. Figure 1(a) illustrates the fan employed in the study and also the experimental setup that is utilized in Figure 1(b). For all experiments the fan rotates at 9,000RPM and is parallel to the thin foil at a distance of 5mm, resulting in flow impinging directly on the foil. The IR camera detects a temperature distribution, which may then be converted to a local heat transfer coefficient, which is of interest to the thermal designer.
The work considers three limiting conditions of motor support designs on a commercially available small scale fan. Firstly, the motor supports are placed at the flow exit side of the fan, which is the most common in electronics cooling; secondly the motor supports are placed at the inlet to the fan and finally a fan without any motor supports and powered by an auxiliary motor is implemented. In the latter the fan and housing are identical to figure 1(a) with motor supports removed.
RESULTS
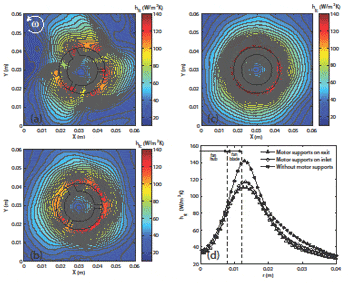
Figure 2(a) to (d) shows the calculated heat transfer coefficients from the measured temperature fields using the setup of Figure 1. Case (a) demonstrates the influence of the motor supports at the exit of the commercially available fan when used as intended by the manufacturer. In this case six zones are clearly identified caused by the interaction between the exit flow and the motor supports. Interestingly, the peak heat transfer coefficient of these six zones is ~ 130W/m2K, while on the same circumferential radius the heat transfer coefficient reaches values of ~ 60 W/m2K. Directly under the motor the heat transfer coefficient is further reduced. This indicates the importance of placement of motor supports relative to any particular location that needs to be aggressively cooled. Case (b) shows the influence of placing the same motor support structure at the fan inlet. Similar to case (a) high heat transfer zones are identifiable, but a more uniform annular type heat transfer coefficient is evident. Case (c) illustrates the resultant local heat transfer coefficient when the motor supports are removed, and a well ordered annular type jet flow is evident, which is symmetric about any line drawn through the centre. Moreover, the peak heat transfer coefficient is now ~ 140W/m2K.
The circumferentially averaged local heat transfer coefficients of cases (a), (b) and (c) are shown in Figure 2(d). The influence of the motor supports on this averaged heat transfer is dramatic, where a 20% increase is measured in the region of the annular jet. Although the gains of 20% may not be possible due the practical necessity of requiring some motor supports for this type of fan arrangement, reducing the size and placing the supports at the inlet provides some improvement in the heat transfer coefficient. Such an approach would allow any given fan to run at a lower speed resulting in increase reliability combined with reduced power consumption and acoustic levels for the same cooling requirements. Alternatively, it could allow higher heat dissipation for the same package temperature when operating at the same speed. Hence this is a simple, cost effective approach, to improving the thermal management of devices where significant gains are attainable for minimal effort.
CONCLUSIONS
Although the influence of motor supports on the thermal performance of an axial flow fan is rarely considered, it is shown here to be important, with heat transfer coefficients increasing by up to 20% for different designs. Placing the motor supports at the inlets provided a small enhancement in heat transfer coefficient, while removing the motor supports completely resulted in greatly improved performance. Therefore the motor supports should be kept as small as possible and placed at the inlet of the fan to provide enhanced thermal performance. Furthermore, this result should be taken into account when designing heat sinks with an impingement cooling methodology, as it indicates that the design of a repeating fin matrix at the exit of an axial flow fan may not be optimum. Further work linking the fan designs and heat sink cooling surfaces needs to be undertaken.
REFERENCES
M. Miyahara, “Small fans for cooling small electronic devices,” Electronics Cooling, vol. 15, pp. 30-32, 2009.
E. J. Walsh, P. A. Walsh, J. Punch, and R. Grimes, “Acoustic emissions from active cooling solutions for portable devices,” IEEE Transactions on Components and Packaging Technologies, vol. 32, pp. 776-783, 2009.
P.A. Walsh, V. Egan, R. Grimes, and E. J. Walsh, “Profile scaling of miniature centrifugal fans,” Heat Transfer Engineering, vol. 30 pp. 130-137, 2009.
V. Egan, J. Stafford, P. Walsh, E. Walsh, and R. Grimes, “An experimental study on the performance of miniature heat sinks for forced convection air cooling,” ASME J. of Heat Transfer, vol. 130, 2009.
J. Stafford, E. J. Walsh, and V. Egan, “Characterizing convective heat transfer using quantitative heated-thin-foil thermography,” Measurement Science and Technology, vol. 20, pp. 11, 2009.
Contact Ed J. Walsh at edmond.walsh@ul.ie.




